A preliminary glance at how derivative contracts are priced. Firstly, it is introduced by the concept of arbitrage.
Arbitrage occurs when equivalent assets or combinations of assets sell for two different prices. This situation creates an opportunity to profit at no risk with no commitment of money.
For example, let’s start with the simplest (and least likely) opportunity for arbitrage – the case of a stock selling for more than one price at a given time.
Assume that a stock is trading in two markets simultaneously. Suppose the stock is trading at $100 in one market and $98 in the other market.
An arbitrager simply buys a share for $98 in one market and immediately sells it for $100 in the other. The arbitrager has no net position in the stock, so it does not matter what price the stock moves to.
He makes an easy $2 at no risk, and we did not have to put up any funds of our own. The sale of the stock at $100 was more than adequate to finance the purchase of the stock at $98.
Naturally, many market participants would do this, which would create downward pressure on the price of the stock in the market where it trades for $100 and upward pressure on the price of the stock in the market where it trades for $98.
Eventually, the two prices must come together so that there is but a single price for the stock. Accordingly, the principle that no arbitrage opportunities should be available is often referred to as the “law of one price”.
An asset can potentially trade in different geographic markets and, therefore, have several spot prices.
This potential would appear to violate the law of one price, but in reality, the law is still upheld. A given asset selling in two different locations is not necessarily the same asset.
If a buyer in one location discovered that it is possible to buy the asset more cheaply in another location, the buyer would still have to incur the cost of moving the asset to the buyer’s location.
Transportation costs could offset any such price differences.
Another Situation in Derivative Pricing
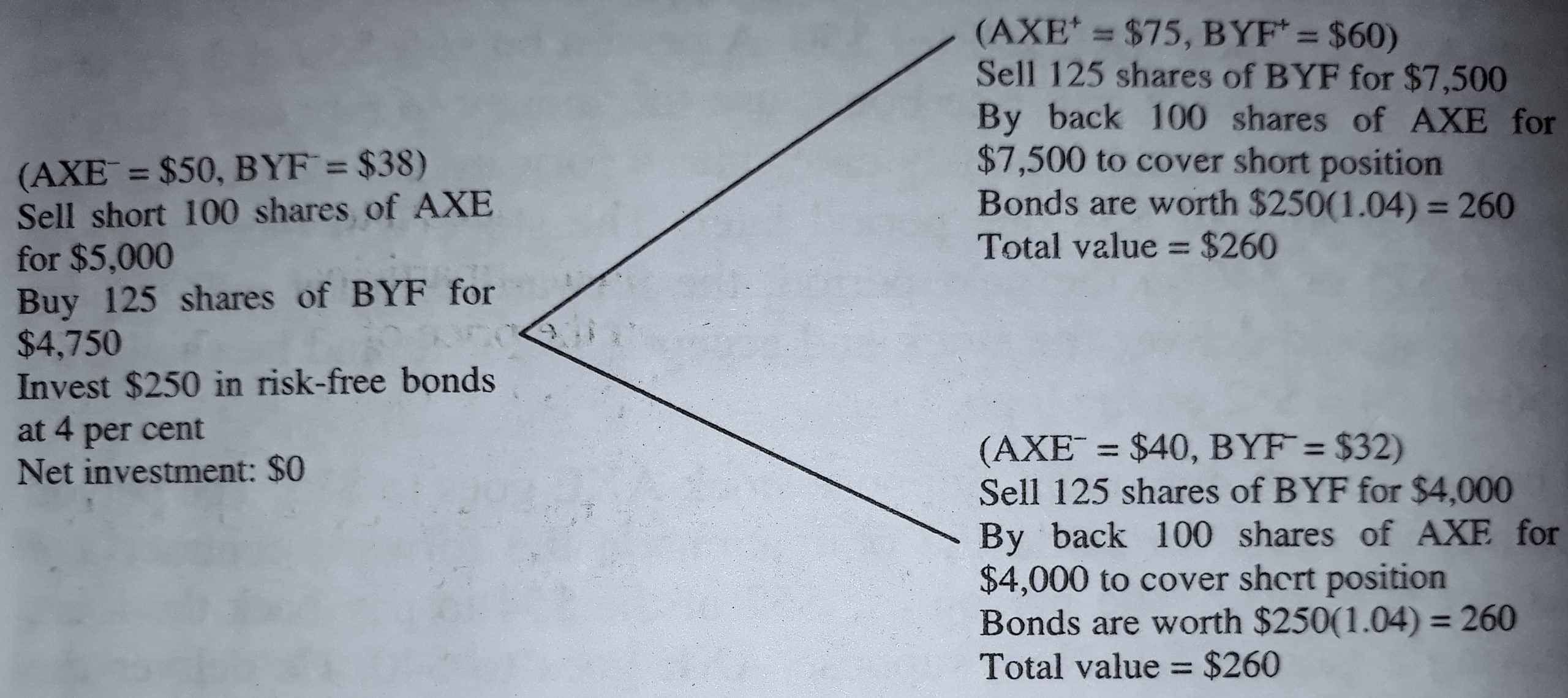
Now suppose we face the situation illustrated in the figure given below. Observe that it has one stock, AXE Electronics, which today is worth $50 and which, one period later, will be worth either $75 or $40:
By denoting these prices as AXE = 50, AXE+ = 75, and AXE~ = 40. Another stock, BYF Technology, is today worth $38, and one period later will be worth $60 or $32.
Thus, BYF = 38, BYF+ = 60, and BYF- = 32. Let us assume the risk-free borrowing and lending rate is 4 percent.
Assume no dividends on either stock during the period covered by this example.
The opportunity exists to make a profit at no risk without committing any of our funds, as demonstrated in the figure given below.
Borrowing 100 shares of stock AXE, which is selling for $50, and sell short, thereby receiving $5,000. Let’s take $4,750 and purchase 125 shares of stock BYF.
By investing the remaining $250 in risk-free bonds at 4 percent. This transaction will not require us to put up any funds of our own – The short sale will be sufficient to fund the investment in BYF and leave money to invest in risk-free bonds.
If the top outcome occurs, sell the 125 shares of BYF for 125 x $60 = $7,500. This amount is sufficient to buy back the 100 shares of $250 x 1.04 = $260.
If the bottom outcome occurs, sell the 125 shares of BYF for 125 x $32 = $4,000 – enough money to buy back the 100 shares of AXE, which is selling for $40.
Again, we will have risk-free bonds worth $260. Regardless of the outcome, we end up with $260.
By putting up no money of our own and ended up with a sure $260. It should be apparent that this is an extremely attractive transaction so that everyone would do it.
The combined actions of multiple investors would drive down the price of AXE and/or drive up the price of BYF until an equilibrium was reached at which this transaction would not be profitable.
Assuming stock BYF’s price remained constant, stock AXE would fall to $47.50. Or assuming stock AXE’s price remained constant, stock BYF would rise to $40.
Of course, this example is extremely simplified. Clearly, a stock price can change to more than two other prices. Also, if a given stock is at one price, another stock may be at any other price.
We have created a simple case here to illustrate a point. When derivatives are involved, the simplification here is relatively safe. In fact, it is quite appropriate.
Arbitrage Opportunity by forwarding Contract
By looking at another type of arbitrage opportunity, which involves a forward contract and will establish an appropriate price for the forward contract.
Let stock AXE sell for $50. A person borrows $50 at 4 percent interest by issuing a risk-free bond, uses the money to buy one share of stock AXE, and simultaneously enters into a forward contract to sell this share at a price of $54 one period later.
The stock will then move to either $75 or $40 in the next period; the forward contract will require that he should deliver the stock and accept $54 for it, and he shall owe $50 x 1.04 = $52 on loan.
Look at the two outcomes. Suppose stock AXE goes to $75. The person delivers the stock to settle the obligation on the forward contract and receives $54 for it.
The person uses $52 of the $54 to pay back the loan, leaving a gain of $2. Now suppose AXE goes to $40.
He delivers the stock, fulfilling the obligation of the forward contract, and receives $54. Again, he uses $52 of the $54 to pay back the loan, leaving a gain of $2.
In either case, the person made $2, free and clear. In fact, he can even accommodate the possibility of more than two future prices for AXE.
The key point is that he faced no risk and did not have to put up any of our own money, but he ended up with $2 – clearly a good deal. In fact, this is what he would call an arbitrage profit But from where did it originate?
It turns out that the forward price the person received, $54, was an inappropriate price given current market conditions.
In fact, it was just an arbitrary price, made up to illustrate the point. To eliminate the opportunity to earn the $2 profit, the forward price should be $52 – equal, not coincidentally, to the amount owed on loan.
It is also no coincidence that $52 is the asset’s price increased by the interest rate.
In this example, many market participants would do this transaction as long as it generates an arbitrage profit.
These forces of arbitrage would either force the forward price down or force the price of the stock up until an equilibrium is reached that eliminates the opportunity to profit at no risk with no commitment of one’s own funds.
We have just had a taste of not only the powerful forces of arbitrage but also a pricing model for one derivative, the forward contract.
In this simple example, according to the pricing model, the forward price should be the spot price increased by the interest rate.
Although there is a lot more to derivative pricing than shown here, the basic principle remains the same regardless of the type of instrument or the complexity of the setting.
Prices are set to eliminate the opportunity to profit at no risk with no commitment of one’s own funds. There are no opportunities for arbitrage profits.
Arbitrage Transactions are Complex
We must acknowledge that there is a large industry of arbitrageurs. So how can such an industry exist if there are no opportunities for riskless profit?
One explanation is that most arbitrage transactions are more complex than this simple example and involve estimating information, which can result in differing opinions.
Arbitrage involving options, e.g., usually requires estimates of a stock’s volatility. Different participants have different opinions about this volatility.
It is quite possible that two counterparties trading with each other can believe that each is arbitraging against the other.
But more importantly, the absence of arbitrage opportunities is upheld, ironically, only if participants believe that arbitrage opportunities do exist.
If market traders believe that no opportunities exist to earn arbitrage profits, then they will not follow market prices and compare these prices with what they ought to be, as in the forward contract.
Without participants watching closely, prices would surely get out of line and offer arbitrage opportunities. Thus, eliminating arbitrage opportunities requires that participants be vigilant to arbitrage opportunities.
In other words, strange as it may sound, disbelief and scepticism concerning the absence of arbitrage opportunities are required in order that it holds as a legitimate principle.
Markets in which arbitrage opportunities are either non-existent or are quickly eliminated are relatively efficient markets.
The study of portfolio theory and investment analysis that efficient markets are those in which it is not possible, except by chance, to earn returns in excess of those that would be fair compensation for the risk assumed.
Although abnormal returns can be earned in a variety of ways, arbitrage profits are definitely examples of abnormal returns, relatively obvious to identify and easy to capture.
Thus, they are the most egregious violations of the principle of market efficiency. A market in which arbitrage profits do not exist is one in which the most obvious violations of market efficiency have been eliminated.
Arbitrage Opportunities Never Exist
Throughout this study session, we shall study derivatives by using the principle of arbitrage as a guide. We will assume that arbitrage opportunities cannot exist for any significant length of time.
Thus, prices must conform to models that assume no arbitrage. On the other hand, we do not want to take the absence of arbitrage opportunities so seriously that we give up and believe that arbitrage opportunities never exist.
Otherwise, they will arise, and someone else will take them from us.